Question 1:
Compare and contrast geostationary and non-geostationary satellites.
Answer:
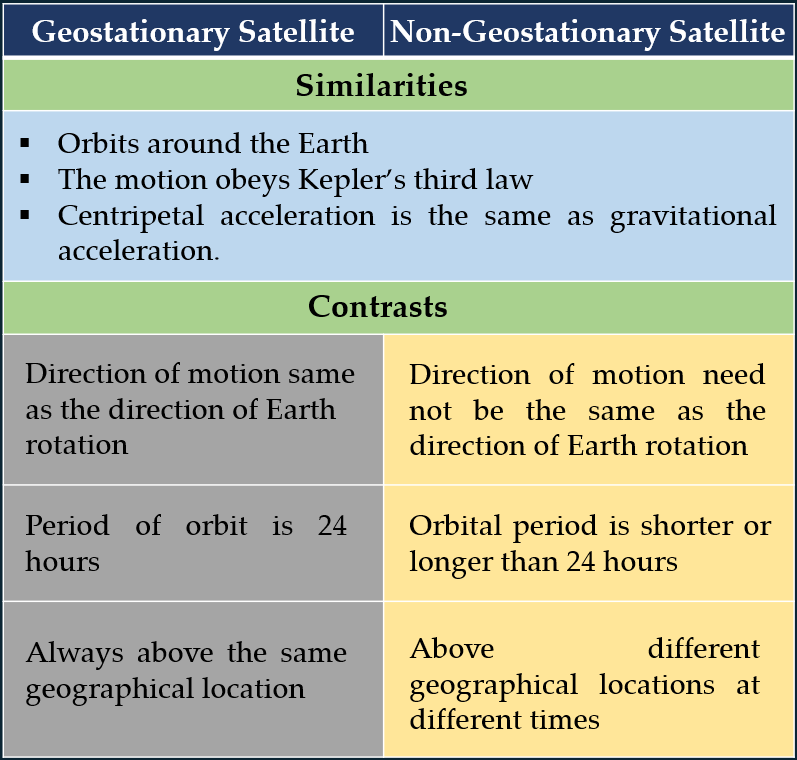
Compare and contrast geostationary and non-geostationary satellites.
Answer:

Question 2:
What factors determine the linear speed of satellites orbiting the Earth?
Answer:
$$ \text { Linear speed of satellite, } v=\sqrt{\frac{G M}{r}} $$
As GM is constant, linear speed of the satellite only depends on the radius of its orbit. If a satellite is at a height, h above the surface of the Earth, r = R + h (R = radius of the Earth).
What factors determine the linear speed of satellites orbiting the Earth?
Answer:
$$ \text { Linear speed of satellite, } v=\sqrt{\frac{G M}{r}} $$
As GM is constant, linear speed of the satellite only depends on the radius of its orbit. If a satellite is at a height, h above the surface of the Earth, r = R + h (R = radius of the Earth).
Question 3:
State two factors which infl uence the value of escape velocity from a planet.
Answer:
$$ \text { Escape velocity, } v=\sqrt{\frac{2 G M}{r}} $$
Thus, the two factors which influence the value of escape velocity from a planet are:
1. Mass of the planet
2. Distance from the centre of the planet
State two factors which infl uence the value of escape velocity from a planet.
Answer:
$$ \text { Escape velocity, } v=\sqrt{\frac{2 G M}{r}} $$
Thus, the two factors which influence the value of escape velocity from a planet are:
1. Mass of the planet
2. Distance from the centre of the planet
Question 4:
Discuss whether escape velocity from the Earth for spacecraft X of mass 1 500 kg is different from spacecraft Y of mass 2 000 kg.
Answer:
No, because the escape velocity, v of an object does not depend on the mass of the object.
Discuss whether escape velocity from the Earth for spacecraft X of mass 1 500 kg is different from spacecraft Y of mass 2 000 kg.
Answer:
No, because the escape velocity, v of an object does not depend on the mass of the object.
Question 5:
Proba-1 satellite orbits the Earth at a height of 700 km. What is the linear speed of this satellite?
[G = 6.67 × 10–11 N m2 kg–2, mass of the Earth = 5.97 × 1024 kg, radius of the Earth = 6.37 × 106 m]
Answer:
$$ \begin{aligned} h & =700 \mathrm{~km}=7.0 \times 10^5 \mathrm{~m} \\ G & =6.67 \times 10^{-11} \mathrm{Nm}^2 \mathrm{~kg}^{-2} \\ M_E & =5.97 \times 10^{24} \mathrm{~kg} \\ R & =6.37 \times 10^6 \mathrm{~m} \\ v & =? \\ r & =R+h=\left(6.37 \times 10^6\right)+\left(7.0 \times 10^5\right) \\ & =7.07 \times 10^6 \mathrm{~m} \end{aligned} $$
$$ \begin{aligned} v & =\sqrt{\frac{G M}{R+h}} \\ & =\sqrt{\frac{\left(6.67 \times 10^{-11} \times\left(5.97 \times 10^{24}\right)\right.}{7.07 \times 10^6}} \\ v & =7504.83 \mathrm{~ms}^{-1} \end{aligned} $$
Proba-1 satellite orbits the Earth at a height of 700 km. What is the linear speed of this satellite?
[G = 6.67 × 10–11 N m2 kg–2, mass of the Earth = 5.97 × 1024 kg, radius of the Earth = 6.37 × 106 m]
Answer:
$$ \begin{aligned} h & =700 \mathrm{~km}=7.0 \times 10^5 \mathrm{~m} \\ G & =6.67 \times 10^{-11} \mathrm{Nm}^2 \mathrm{~kg}^{-2} \\ M_E & =5.97 \times 10^{24} \mathrm{~kg} \\ R & =6.37 \times 10^6 \mathrm{~m} \\ v & =? \\ r & =R+h=\left(6.37 \times 10^6\right)+\left(7.0 \times 10^5\right) \\ & =7.07 \times 10^6 \mathrm{~m} \end{aligned} $$
$$ \begin{aligned} v & =\sqrt{\frac{G M}{R+h}} \\ & =\sqrt{\frac{\left(6.67 \times 10^{-11} \times\left(5.97 \times 10^{24}\right)\right.}{7.07 \times 10^6}} \\ v & =7504.83 \mathrm{~ms}^{-1} \end{aligned} $$
