Question 1:

Figure 4.23 shows an electric steamer. Explain how the fish is heated.
Answer:
– Water has large specific latent heat of vaporization.
– When the steam condenses on the fish, the specific latent heat is released directly to the fish.
– This enable the fish to be cooked faster.

Figure 4.23 shows an electric steamer. Explain how the fish is heated.
Answer:
– Water has large specific latent heat of vaporization.
– When the steam condenses on the fish, the specific latent heat is released directly to the fish.
– This enable the fish to be cooked faster.
Question 2:
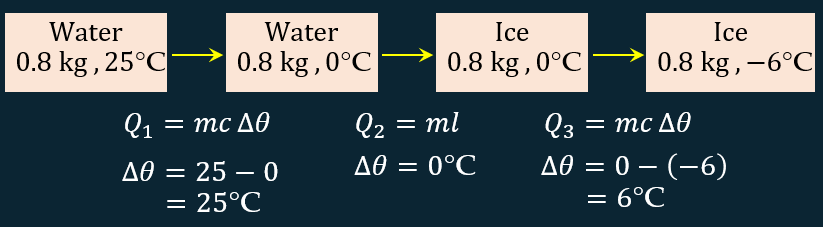
What is the amount of heat released when 0.8 kg of water at 25°C cools until it becomes ice at –6°C? State the assumptions you make in your calculations.
[Specific heat capacity of water, cwater = 4.2 × 103 J kg–1 °C–1, specific heat capacity of ice, cice = 2.0 × 103 J kg–1 °C–1 and specific latent heat of fusion of ice, lf = 3.34 × 105 J kg–1]
Answer:
$$ \begin{aligned} c_{\text {water }} & =4200 \mathrm{~J} \mathrm{~kg}^{-1} \mathrm{C}^{-1} \\ c_{\text {ice }} & =2.0 \times 10^3 \mathrm{~J} \mathrm{~kg}^{-1} \mathrm{C}^{-1} \\ l_{\mathrm{f}} & =3.34 \times 10^5 \mathrm{~J} \mathrm{~kg}^{-1} \end{aligned} $$
Amount of heat supplied, $$ \begin{aligned} Q= & Q_1+Q_2+Q_3 \\ = & m c \Delta \theta+m l+m c \Delta \theta \\ = & \left(0.8 \times 4.2 \times 10^3 \times 25\right)+\left(0.8 \times 3.34 \times 10^5\right)+ \\ & \left(0.8 \times 2.0 \times 10^3 \times 6\right) \\ Q= & 360800 \mathrm{~J} \end{aligned} $$
What is the amount of heat released when 0.8 kg of water at 25°C cools until it becomes ice at –6°C? State the assumptions you make in your calculations.
[Specific heat capacity of water, cwater = 4.2 × 103 J kg–1 °C–1, specific heat capacity of ice, cice = 2.0 × 103 J kg–1 °C–1 and specific latent heat of fusion of ice, lf = 3.34 × 105 J kg–1]
Answer:

$$ \begin{aligned} c_{\text {water }} & =4200 \mathrm{~J} \mathrm{~kg}^{-1} \mathrm{C}^{-1} \\ c_{\text {ice }} & =2.0 \times 10^3 \mathrm{~J} \mathrm{~kg}^{-1} \mathrm{C}^{-1} \\ l_{\mathrm{f}} & =3.34 \times 10^5 \mathrm{~J} \mathrm{~kg}^{-1} \end{aligned} $$
Amount of heat supplied, $$ \begin{aligned} Q= & Q_1+Q_2+Q_3 \\ = & m c \Delta \theta+m l+m c \Delta \theta \\ = & \left(0.8 \times 4.2 \times 10^3 \times 25\right)+\left(0.8 \times 3.34 \times 10^5\right)+ \\ & \left(0.8 \times 2.0 \times 10^3 \times 6\right) \\ Q= & 360800 \mathrm{~J} \end{aligned} $$
Assumption : Heat released is only from the water but not from the surrounding.
