Question 1:
Diamond is a precious stone that always appears shiny. Critical angle of diamond in air is 24°.
(a) (i) What is the meaning of critical angle?
(ii) Determine the value of refractive index of diamond.
(b) Figure 1 shows three diamonds of different shapes. A ray enters each diamond as shown in Figure 1. Complete the path of light rays until the ray emerges again into air in Figure 1.
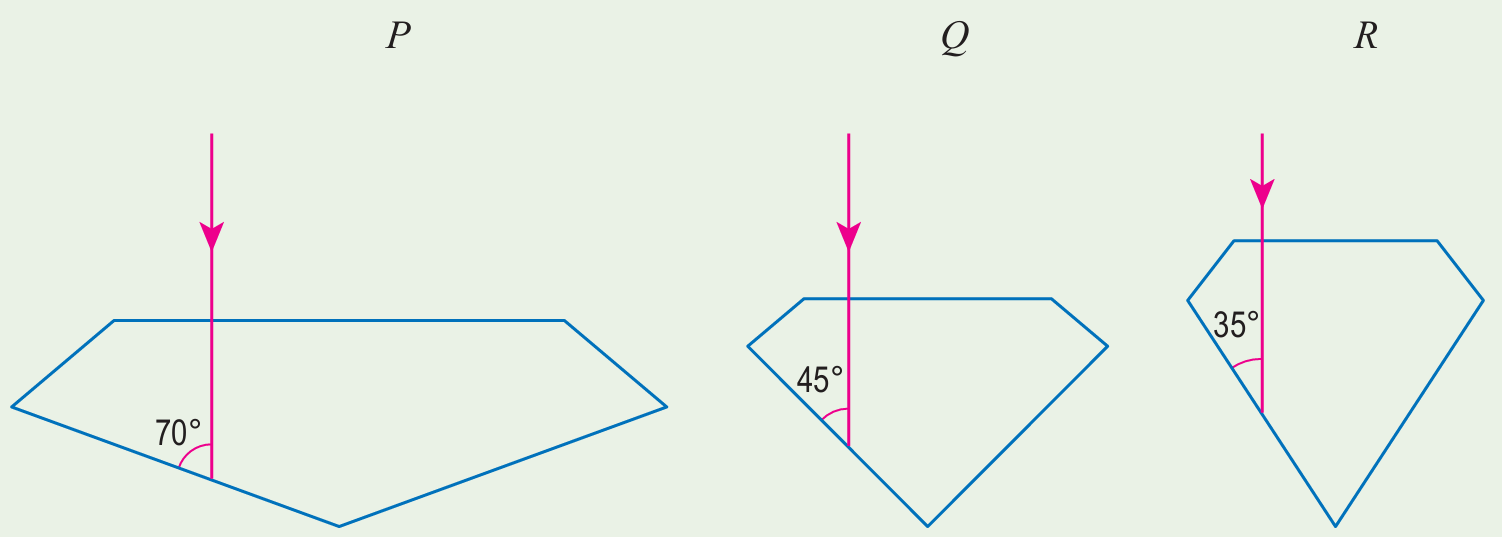
(c) State the physics concepts involved in this phenomenon.
Answer:
(a)(i) Critical angle is the angle of incidence in an optically denser medium when the angle of refraction is 90o.
(a)(ii)
$$ \begin{aligned} n & =\frac{1}{\sin c} \\ & =\frac{1}{\sin 24^{\circ}} \\ & =2.46 \end{aligned} $$
(b)
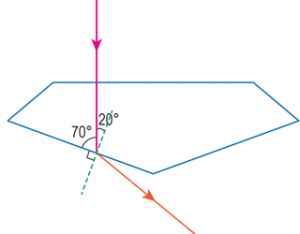

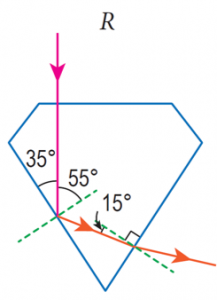
(c) Total internal reflection and refraction of light.
Diamond is a precious stone that always appears shiny. Critical angle of diamond in air is 24°.
(a) (i) What is the meaning of critical angle?
(ii) Determine the value of refractive index of diamond.
(b) Figure 1 shows three diamonds of different shapes. A ray enters each diamond as shown in Figure 1. Complete the path of light rays until the ray emerges again into air in Figure 1.

(c) State the physics concepts involved in this phenomenon.
Answer:
(a)(i) Critical angle is the angle of incidence in an optically denser medium when the angle of refraction is 90o.
(a)(ii)
$$ \begin{aligned} n & =\frac{1}{\sin c} \\ & =\frac{1}{\sin 24^{\circ}} \\ & =2.46 \end{aligned} $$
(b)



(c) Total internal reflection and refraction of light.
Question 2:
Figure 2 shows a glass block with a refractive index of 1.50 placed between the eyes of the observer, E and the object P. If the thickness of the glass is 30.0 cm, what is the distance between object P and its image?

Answer:
$$ \begin{aligned} n & =\frac{\text { real depth }}{\text { apparent depth }}=\frac{H}{h} \\ h & =\frac{H}{n} \\ & =\frac{30}{1.5} \\ & =20 \mathrm{~cm} \end{aligned} $$
Distance between object P and its image
= 30 – 20
= 10 cm
Figure 2 shows a glass block with a refractive index of 1.50 placed between the eyes of the observer, E and the object P. If the thickness of the glass is 30.0 cm, what is the distance between object P and its image?

Answer:
$$ \begin{aligned} n & =\frac{\text { real depth }}{\text { apparent depth }}=\frac{H}{h} \\ h & =\frac{H}{n} \\ & =\frac{30}{1.5} \\ & =20 \mathrm{~cm} \end{aligned} $$
Distance between object P and its image
= 30 – 20
= 10 cm
