Question 3:
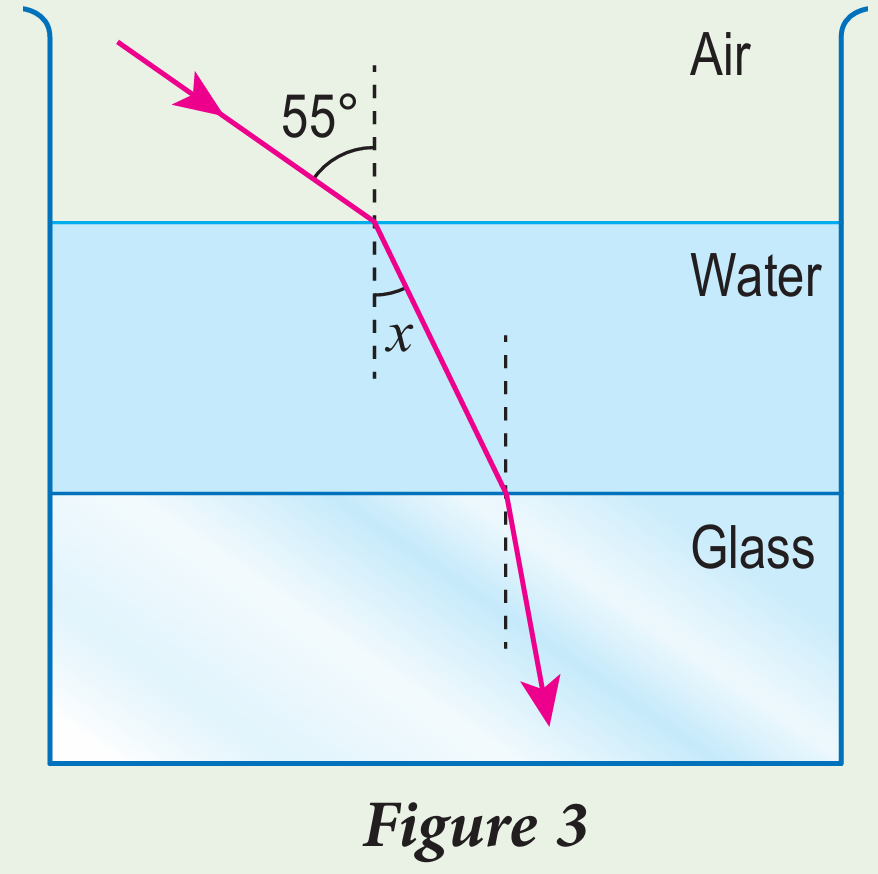
Figure 3 shows a light ray travelling from air to water and then entering a glass block. The refractive index of water is 1.33.
(a) Determine angle x.
(b) If speed of light in air is 3.0 × 108 m s−1, what is the speed of light in water?
(c) Between water and glass, which medium has a higher optical density? Explain your answer based on Figure 3.
Answer:
(a)
$$ \begin{aligned} n & =\frac{\sin i}{\sin r} \\ 1.33 & =\frac{\sin 55^{\circ}}{\sin x} \\ \sin x & =\frac{\sin 55^{\circ}}{1.33} \\ x & =38.02^{\circ} \end{aligned} $$
(b)
$$ \begin{aligned} & \text { Refractive index, } n=\frac{\text { speed of light in vacuum }}{\text { speed of light in medium }} \\ & n=\frac{c}{v} \\ & \text { Speed of light in water }=\frac{c}{n} \\ & =\frac{3.0 \times 10^8}{1.33} \\ & =2.26 \times 10^8 \mathrm{~m} \mathrm{~s}^{-1} \end{aligned} $$
(c)
Glass has a higher optical density because when light travels from water to glass, the light is refracted towards the normal.

Figure 3 shows a light ray travelling from air to water and then entering a glass block. The refractive index of water is 1.33.
(a) Determine angle x.
(b) If speed of light in air is 3.0 × 108 m s−1, what is the speed of light in water?
(c) Between water and glass, which medium has a higher optical density? Explain your answer based on Figure 3.
Answer:
(a)
$$ \begin{aligned} n & =\frac{\sin i}{\sin r} \\ 1.33 & =\frac{\sin 55^{\circ}}{\sin x} \\ \sin x & =\frac{\sin 55^{\circ}}{1.33} \\ x & =38.02^{\circ} \end{aligned} $$
(b)
$$ \begin{aligned} & \text { Refractive index, } n=\frac{\text { speed of light in vacuum }}{\text { speed of light in medium }} \\ & n=\frac{c}{v} \\ & \text { Speed of light in water }=\frac{c}{n} \\ & =\frac{3.0 \times 10^8}{1.33} \\ & =2.26 \times 10^8 \mathrm{~m} \mathrm{~s}^{-1} \end{aligned} $$
(c)
Glass has a higher optical density because when light travels from water to glass, the light is refracted towards the normal.
Question 4:
Mariam carried out an experiment with a semi-circular glass block and a ray box. Figure 4 shows the path of light ray entering the glass block at point R and travelling towards the centre of the semi-circular glass block, point S.
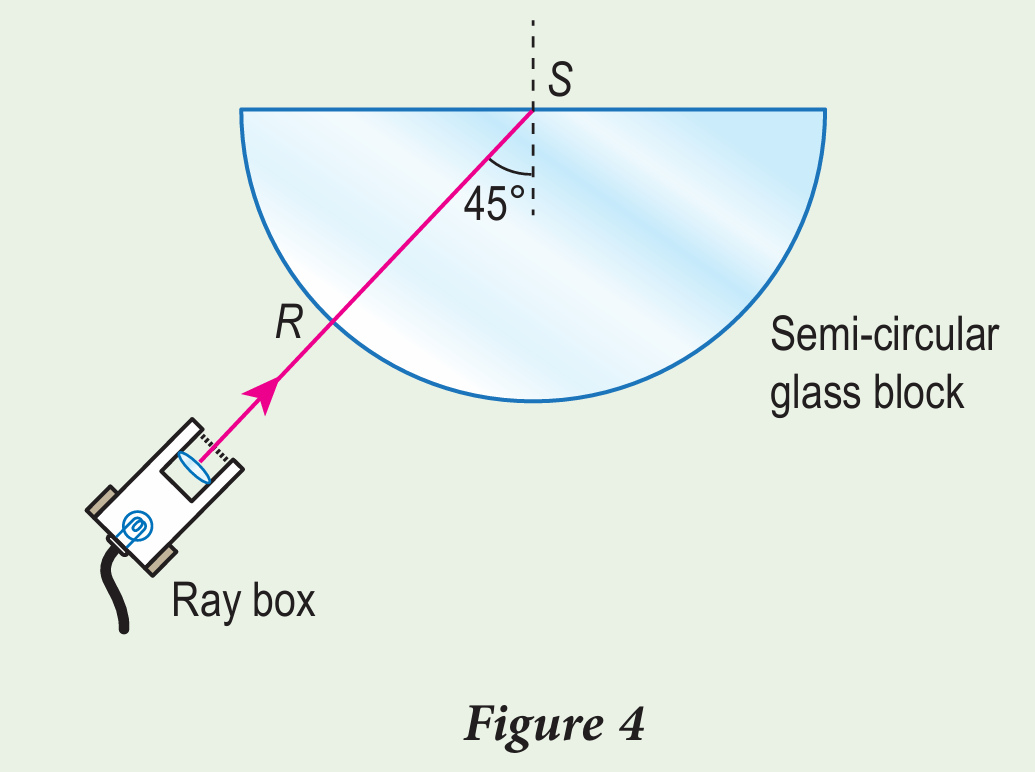
(a) Why does the light ray not change direction at point R?
(b) If the refractive index of the glass block is 1.52, determine the critical angle in this medium.
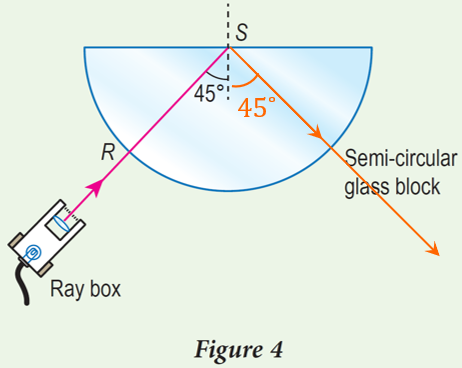
(c) Draw the path of light ray aft er point S and mark the value of the angle of the light ray with the normal at point S.
Answer:
(a)
RS is the radius of a semi-circle and is the normal to the incident ray at point R, i = 0 so r = 0
(b)
$$ \begin{aligned} \sin c & =\frac{1}{n} \\ \sin c & =\frac{1}{1.52} \\ c & =41.14^{\circ} \end{aligned} $$
(c)
Mariam carried out an experiment with a semi-circular glass block and a ray box. Figure 4 shows the path of light ray entering the glass block at point R and travelling towards the centre of the semi-circular glass block, point S.

(a) Why does the light ray not change direction at point R?
(b) If the refractive index of the glass block is 1.52, determine the critical angle in this medium.
(c) Draw the path of light ray aft er point S and mark the value of the angle of the light ray with the normal at point S.
Answer:
(a)
RS is the radius of a semi-circle and is the normal to the incident ray at point R, i = 0 so r = 0
(b)
$$ \begin{aligned} \sin c & =\frac{1}{n} \\ \sin c & =\frac{1}{1.52} \\ c & =41.14^{\circ} \end{aligned} $$
(c)

